Coherence condition of two proofs in Cubical Agda?
In Cubical Agda, there are multiple ways to prove a property of paths. For example, the most straightforward way to prove symmetricity is to use the primitive operator ~ on intervals:
≡-symm : ∀ {ℓ} {A : Type ℓ} {a b : A} → a ≡ b → b ≡ a
≡-symm {ℓ}{A}{a}{b} p = λ i → p (~ i)
But there are at least two more ways to prove the same thing. One you may consider is to use hcomp, and set two egdes of a square (2-d cube) to refl:
≡-symm₁ ≡-symm₂ ≡-symm₃ : ∀ {ℓ} {A : Type ℓ} {a b : A}
→ a ≡ b → b ≡ a
≡-symm₁ {ℓ}{A}{a}{b} p i = hcomp walls a
where
walls : ∀ j → Partial (∂ i) A
walls j (i = i0) = p j
walls j (i = i1) = a
≡-symm₂ {ℓ}{A}{a}{b} p i = hcomp walls (p i)
where
walls : ∀ j → Partial (∂ i) A
walls j (i = i0) = p j
walls j (i = i1) = p (~ j)
≡-symm₃ {ℓ}{A}{a}{b} p i = hcomp walls b
where
walls : ∀ j → Partial (∂ i) A
walls j (i = i0) = b
walls j (i = i1) = p (~ j)
You may also use coercion. The key is to construct a type line from refl : a ≡ a to something of type b ≡ a. Basically, you need to fix the right endpoint of a path, and let the left endpoint “slide” along some known path, and here it is p : a ≡ b:
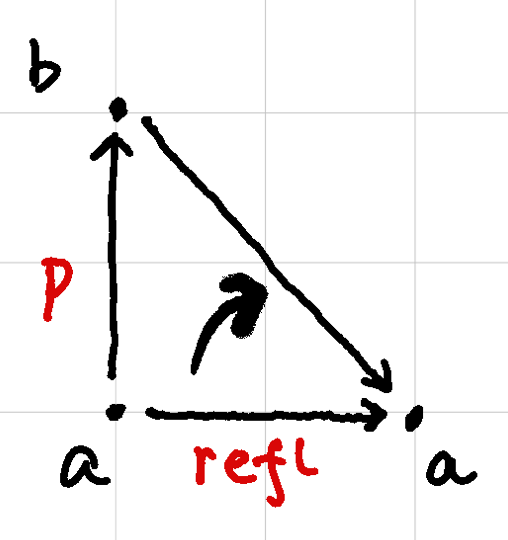
coe0→1 : ∀ {ℓ} (A : I → Type ℓ) → A i0 → A i1
coe0→1 A a = transp (λ i → A i) i0 a
≡-symm : ∀ {ℓ} {A : Type ℓ} {a b : A} → a ≡ b → b ≡ a
≡-symm {ℓ}{A}{a}{b} p = coe0→1 (λ i → p i ≡ a) refl
Now we have many syntactically different proofs of path symmetricity, can we ask what is the coherence condition of where these proofs are considered “equivalent”? What about other proofs like path transitivity?
(Continued)
So far we know that:
The CCHM paper shows that
transpcan be expressed as a special case ofcomp.Γ ⊢ (transpⁱ A a) = (compⁱ A [] a) : A(i1)The Cartesian Cubical Type Theory shows
hcomcan be expressed bycoe.The Cubical Agda shows
coeand be implemented withtransp.
To do:
- Prove (1) and (2) in Cubical Agda.